Groundwater Flow Equations (pp. 130-135; XX-XX)¶
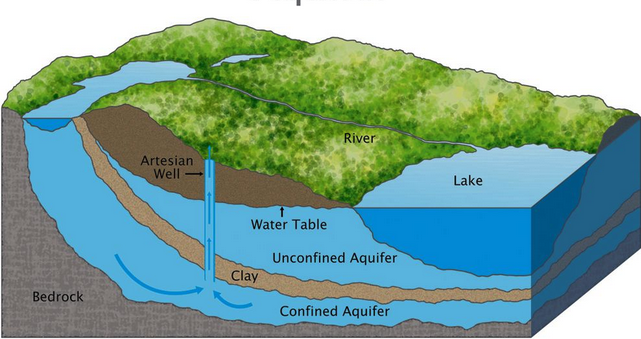
Confined Aquifer¶
\[S \frac{\partial h}{\partial t} = \frac{\partial}{\partial x}(T_x\frac{\partial h}{\partial x})
+ \frac{\partial}{\partial y}(T_y\frac{\partial h}{\partial y}) \pm source/sink \]
Unconfined Aquifer¶
\[S_y \frac{\partial h}{\partial t} = \frac{\partial}{\partial x}(K_x h \frac{\partial h}{\partial x})
+ \frac{\partial}{\partial y}(K_y h \frac{\partial h}{\partial y}) \pm source/sink \]
Solutions (p. 134)¶
Solved by a model that consists of the governing flow equation (one of the above), boundary conditions, and initial conditions. Generally the goal is a description of the head at any location and time.
Solution methods:
Analysis. Some geometries are suitable for analysis (using calculus), other complex geometries are solved using computer programs implementing numerical methods.
Computer Programs