Control Volumes¶
A control volume (CV) is the equivalent of a free-body diagram in other fields of engineering mechanics (statics, dynamics).
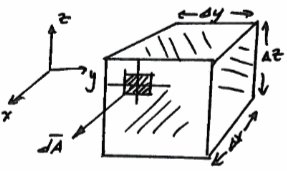
A control volume is some defined area in space, as depicted by the cube below.
Note
The control volume is the basis of reynold’s transport theorem that is employed to allow analysis from a Eulerian reference frame rather than tracking individual particles. The goal is to describe fundamental laws of mechanics in integral form
The bounding surface is called the control surface (CS)
The outward pointing area vectors for each face are shown above; these play an important role in application of CV analysis.
The principle is to express various conservation principles in integral form including:
Conservation of mass (continunity)
¶
\(\frac{dm}{dt}|_{sys} = 0\)
Conservation of linear momentum
¶
\(m\frac{d\bar V}{dt}|_{sys} = \sum \bar F\)
Conservation of angular momentum
¶
\(m\frac{d\bar \omega}{dt}|_{sys} = \sum (\bar r \times \bar F)\)
Conservation of energy
¶
\(\frac{E}{dt}|_{sys} = \frac{dQ}{dt} - \frac{dW}{dt}\)
where \(Q\) is heat flow into the system and \(W\) is work done by the system.
Entropy principle
¶
\(\frac{S}{dt}|_{sys} >= \frac{1}{T}\frac{dQ}{dt}\)
where \(Q\) is heat flow into the system, S is entropy, and T is the absolute temperature of the system.
The first four conservation principles are the most useful in fluid mechanics problems; the last principle is applied in various forms in mechanical and chemical thermodynamics problems - its relevant in Civil and Environmental Engineering, but covered in later classes.